**Unveiling the Mysteries of the Fibonacci Sequence: Nature's Mathematical Marvel**

In the enchanting realm of mathematics, certain patterns and sequences reveal themselves as captivating works of art. One such masterpiece, the Fibonacci Sequence, has fascinated mathematicians, scientists, and artists for centuries. Its ubiquity in the natural world and its intriguing mathematical properties make it a subject worthy of exploration. In this blog post, we'll embark on a journey to unravel the mysteries of the Fibonacci Sequence, from its humble origins to its profound impact on various aspects of life.
**The Birth of a Sequence**
The story of the Fibonacci Sequence begins in the early 13th century with the Italian mathematician Leonardo of Pisa, also known as Fibonacci. While Fibonacci didn't discover the sequence itself, his work "Liber Abaci" introduced it to the Western world. The sequence had actually been previously described in Indian mathematics.
So, what is the Fibonacci Sequence? It is a series of numbers where each number is the sum of the two preceding ones, typically starting with 0 and 1. The sequence looks like this:
0, 1, 1, 2, 3, 5, 8, 13, 21, 34, ...
As you can see, each number is obtained by adding the two numbers immediately before it. This simple rule leads to a cascade of numbers that appears in numerous unexpected places.
**The Golden Ratio: Nature's Aesthetic**
One of the most remarkable properties of the Fibonacci Sequence is its connection to the Golden Ratio. The Golden Ratio, often denoted as φ (phi), is approximately equal to 1.61803398875. This irrational number has an uncanny presence in art, architecture, and nature.

The Golden Ratio manifests when you take consecutive Fibonacci numbers and divide them. As you go further down the sequence, the ratio of successive Fibonacci numbers converges towards the Golden Ratio. This ratio is aesthetically pleasing to the human eye, which is why it appears in art and design.
Architects like Le Corbusier and artists like Salvador Dalí have incorporated the Golden Ratio into their work. From the proportions of the Parthenon in Athens to the spirals of a seashell, the Golden Ratio seems to be an underlying design principle in the natural world.
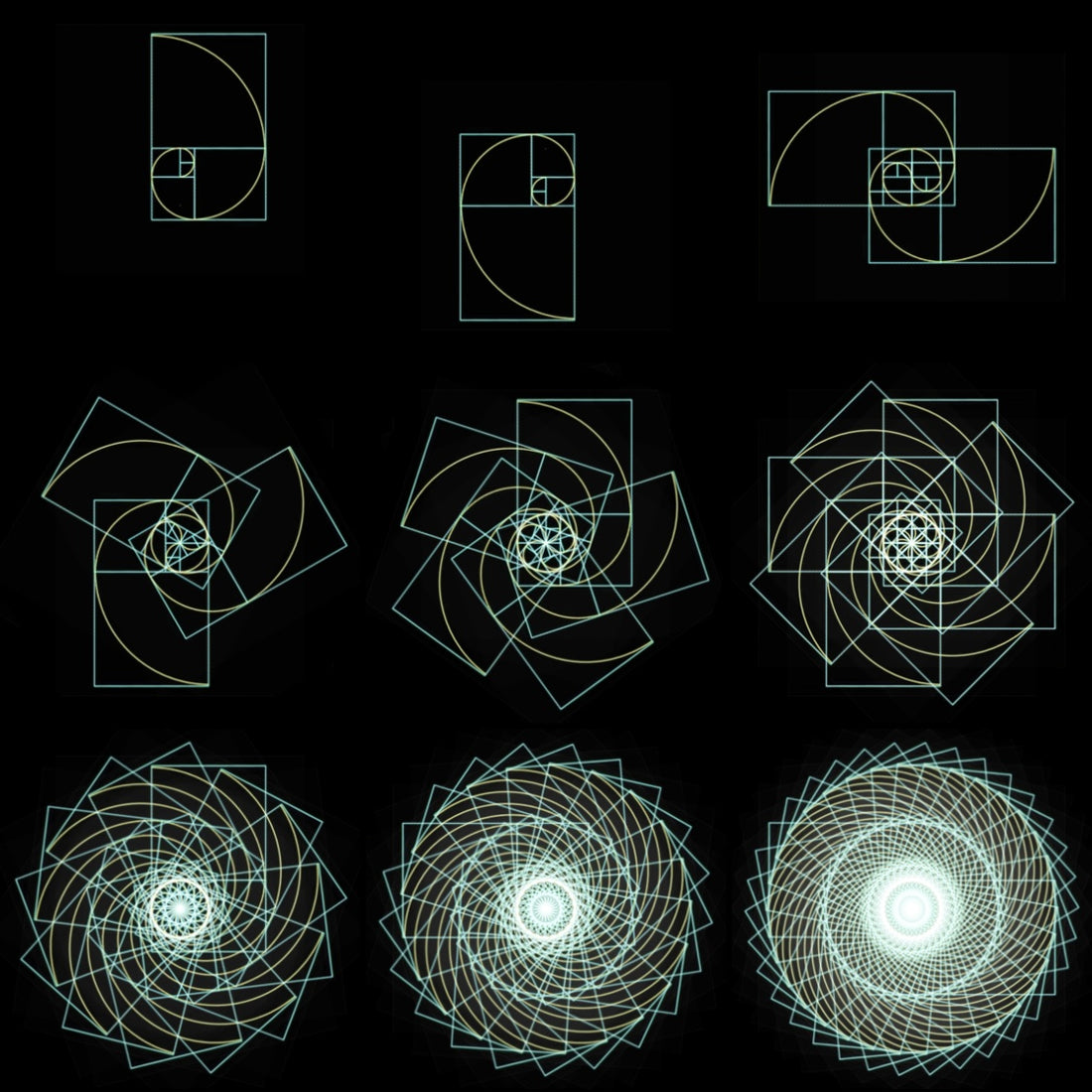
**The Fibonacci Spiral: Nature's Blueprint**
Another captivating aspect of the Fibonacci Sequence is the Fibonacci Spiral. This spiral emerges when you draw squares with sides of Fibonacci numbers and connect them with arcs. The result is a visually stunning spiral that also appears frequently in nature.
For instance, think of a pinecone or a pineapple. The spirals formed by the seeds or scales follow the Fibonacci pattern. The number of spirals typically corresponds to consecutive Fibonacci numbers. This spiral structure allows for efficient packing and optimal space utilization in various natural forms.
https://natureofflowers.com/products/fibonacci-sequence-c-type-print
**Fibonacci in Botany: Phyllotaxis**
In the world of botany, the arrangement of leaves, petals, or seeds on a plant is governed by a phenomenon known as phyllotaxis, and it often follows the Fibonacci sequence. This arrangement ensures that each leaf or flower gets maximum exposure to sunlight and minimizes shading from neighboring structures.
Sunflowers provide a prime example of this. If you examine the seeds in the center of a sunflower, you'll notice two sets of spirals, typically in opposite directions. These spirals follow the Fibonacci pattern, ensuring the most efficient packing of seeds.
**Fibonacci in Art and Music**
The influence of the Fibonacci Sequence extends to the world of art and music as well. In art, artists like Georges Seurat have used the concept of pointillism, where small dots are placed in a pattern following the Fibonacci Spiral, creating stunning visual effects when viewed from a distance.
In music, composers have explored the Fibonacci Sequence in various ways. The famous composer Béla Bartók used the Fibonacci numbers in his compositions to create intricate rhythms and structures that captivate the listener.
**Fibonacci in Mathematics: Beyond the Sequence**
While the Fibonacci Sequence itself is fascinating, its influence reaches far beyond its numbers. It has deep connections to other areas of mathematics. For example, it's related to the Lucas numbers, which are a similar sequence that starts with 2 and 1 instead of 0 and 1. Additionally, it has implications in number theory, combinatorics, and even in algorithms for optimizing financial trading strategies.
**Conclusion: A Mathematical Wonder**
In conclusion, the Fibonacci Sequence is much more than a series of numbers; it's a window into the hidden order of the natural world and a testament to the beauty of mathematics. From its humble beginnings in the works of Fibonacci to its profound impact on art, nature, and mathematics, the Fibonacci Sequence continues to inspire and mystify those who delve into its secrets. Whether you're an artist seeking inspiration, a mathematician exploring its properties, or simply a curious soul marveling at the wonders of nature, the Fibonacci Sequence offers something for everyone to appreciate and ponder.
So, next time you encounter a sunflower, a seashell, or a piece of art that captivates your senses, remember the underlying beauty of the Fibonacci Sequence, quietly orchestrating the elegance of the world around us.
---
I hope you find this blog post informative and enjoyable! If you have any further questions or would like to explore any specific aspect of the Fibonacci Sequence, please feel free to ask.
Do you want to add this sequence to your wardrobe check this out https://natureofflowers.com/products/fibonacci-sequence-softstyle-t-shirt